Optical leaky modes
Computation of the leaky modes (resonances) of optical devices based on open waveguides with heterogeneous cores
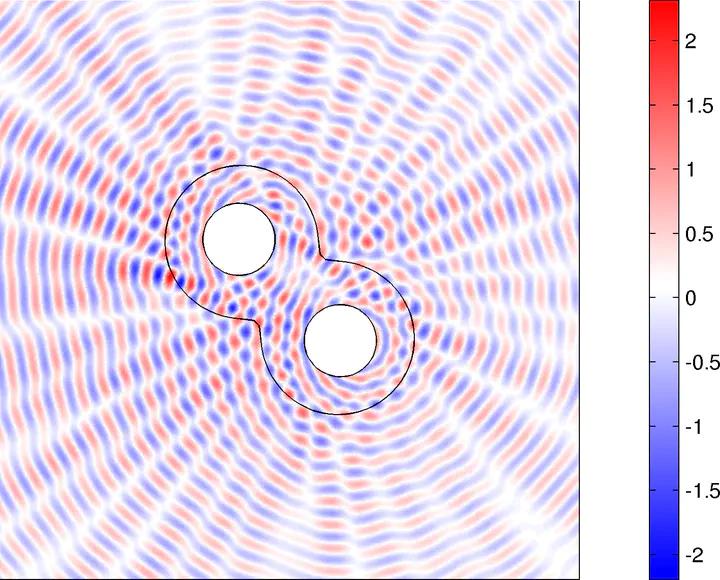 Scattering field in an open waveguide with heterogeneous tranversal section
Scattering field in an open waveguide with heterogeneous tranversal sectionDuring the last decade, several authors have addressed that the PML technique [1] can be used not only for the computation of the near-field in time-dependent and time-harmonic scattering problems, but also to compute numerically the resonances in open cavities. Despite such complex resonances are not natural eigen-frequencies of the physical system, the numerical determination of this kind of eigenvalues provides information about the model, what can be used in further applications. For instance, the computation of leaky modes in an open waveguide is used as the spectral basis for a modal decomposition technique [2] of complex scattering patterns (see Figure on the top).
 Figure 1: Pseudospectra of the discrete non-normal operator
associated to a wave scattering problem truncated with a Perfectly Matched
Layer.
Figure 1: Pseudospectra of the discrete non-normal operator
associated to a wave scattering problem truncated with a Perfectly Matched
Layer.
In the framework of the PML technique, to ensure that the complex eigenvalues are independent of the particular choices of the so-called PML absorption coefficient, the theoretical background is related with the complex scaling technique developed in the seventies by Aguilar and Combes. However, the theoretical results do not guarantee any convergence result on the PML error, which comes from the spurious reflections originated by the truncation of the PML layer to a finite thickness.
Moreover, the numerical computation of these complex resonances have been revealed highly unstable for standard finite element methods (FEM). The numerical results depend strongly not only on the thickness of the PML layer but also on the position of its inner PML boundary with respect to physical domain of the problem. All these numerical difficulties can be interpreted by using the concept of pseudospetrum (see Figure 1) associated to the PML problem and its non-normal behavior (see, for details [3]) .
References
[1] J. P. Bérenger.A perfectly matched layer for the absorption of electromagnetic waves.
Journal of Computational Physics, 114 (1994) 185-200.
[2]
A.-S. Bonnet-BenDhia, B. Goursaud, c. Hazard, A. Prieto.
Finite element computation of leaky modes in stratified waveguides.
In: Ultrasonic Wave Propagation in Non Homogeneous Media, Chapter 7
(A. Leger, M. Deschamps eds.), Springer (2009), 73-86.
[3]
L. N. Trefethen.
Spectra and pseudospectra.
Princeton University Press (2005).